

Posted by: Alesia Netuk
Updated: October 2nd, 2023
The Comprehensive Guide To Number Bonds
The Comprehensive Guide To Number Bonds
Number bonds are used to help children see the relationship between numbers. They help them develop an understanding of how numbers join together and how they are broken apart. Number bonds can be introduced using three steps, with each building on the previous step’s understandings.
What are Number Bonds?
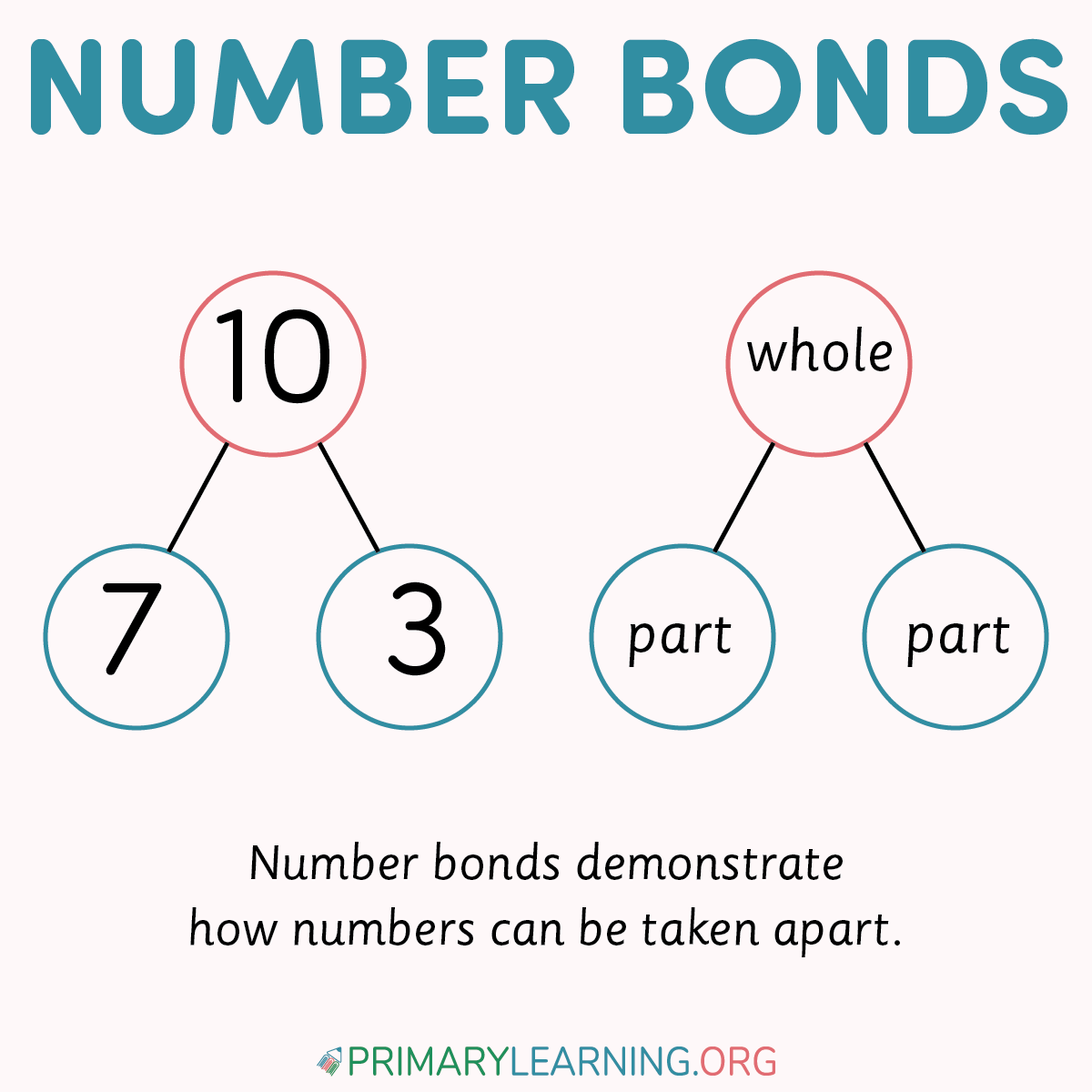
Number bonds show the different ways to create a number. For example, the number five can be created by joining one and four, two and three, or five and zero. Children also learn that these number combinations can be reversed and the same total is achieved. For example, one and four make five and so do four and one.
Number bonds can also be used to demonstrate how numbers can be taken apart. Using the example from above, if children learn that one and four make five, this understanding can be extended to identify that five take away four leaves one.
How to use number bonds for addition
To create number bonds, place the total number in a circle. Then, draw two downward-facing lines, with each connecting to an empty circle. The goal is to determine two numbers that can be combined to create the total number. For example, if the number eight is in the top circle, the bottom circles could each contain a four. (i.e., 4+4=8)
Number bonds can be introduced using totals up to ten. Being able to manipulate and see the relationships between numbers up to ten is a foundational math concept that is important for children to solidify. Understanding numbers to ten is a precursor to working with larger numbers.
Concrete Step
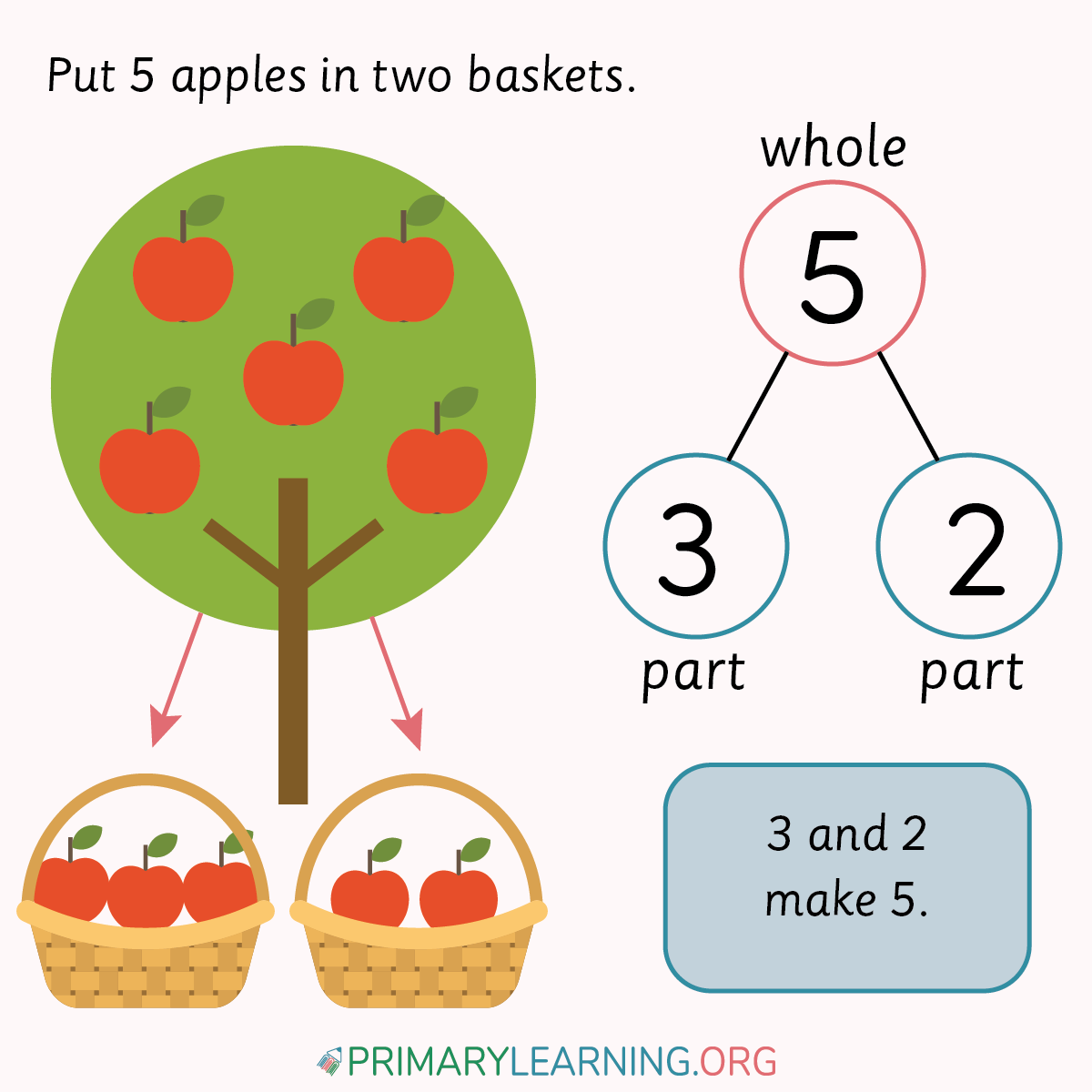
Number bonds can be introduced using three steps: concrete, pictorial, and abstract. The concrete step allows children to use concrete materials to explore counting and number relationships. They can count objects such as counters, plastic chips, gems, or stones. You can also give them a number and ask them to represent it using their objects.
These activities allow children to practice one-to-one correspondence, which is essential for understanding number concepts. It means that they can count objects accurately by counting each one and counting it only once. When children lack this skill, you may see them missing objects or counting them more than once.
Once they have solidified their ability to count objects accurately, children can move on to joining two groups of objects together. For example, they can bring together a group of three counters and a group of two counters to discover that they make five. This provides an introduction to addition.
During the concrete step, children can also explore the concept of subtraction. Have them begin with a certain number of counters. Next, have them take some away. For example, have them create a group of eight counters then remove three. They can discover how they are left with five.
Pictorial Step
During the pictorial step, children move away from using concrete materials to represent numbers. Instead, they use paper or whiteboards to write number bonds. Using the addition example described above, they would write the number five in the top circle and the numbers three and two in the bottom circles, since three and two can be joined to create five.
To introduce this step, have children begin by making the number groups using concrete materials. Next, demonstrate how they can represent their concrete materials on paper or a whiteboard. When they are ready, move away from using concrete materials and go straight to the pictorial representation.
Abstract Step
The abstract step is like the traditional algorithm we use when adding and subtracting. Using the same example as above, the joining of three and two to make five is represented as 3+2=5. Like the pictorial step, these questions can be done on paper or whiteboards with dry-erase markers.
Use caution when moving to the abstract step and make sure children have a thorough understanding of the concrete and pictorial steps first. If children are struggling to understand the algorithms, step back to using pictures or concrete materials to support them.
Number bond addition and subtraction worksheets and printables:
Addition Facts To 10
With this worksbook kindergarten kids practice addition facts to 10. No-prep activity sheets that combine practicing addition facts and hands-on learning.
Addition Facts To 10
Addition With Manipulatives
With this worksbook kindergarten kids practice addition up to 10 using different strategies and manipulatives. Kids use ten frames, number lines, and cubes to solve the addition facts.
Addition With Manipulatives
Cut & Solve – Number Bonds #1
With this worksheet kids solve addition and subtraction problems up to 6. Kids build essential mental math problem-solving skills.
Cut & Solve – Number Bonds #1
Cut & Solve – Number Bonds #2
With this worksheet kids solve addition and subtraction problems up to 7. Kids build essential mental math problem-solving skills.
Cut & Solve – Number Bonds #2
Cut & Solve – Number Bonds #3
With this worksheet kids solve addition and subtraction problems up to 8. Kids build essential mental math problem-solving skills.
Cut & Solve – Number Bonds #3
Cut & Solve – Number Bonds #4
With this worksheet kids solve addition and subtraction problems up to9. Kids build essential mental math problem-solving skills.
Cut & Solve – Number Bonds #4
Cut & Solve – Number Bonds #5
With this worksheet kids solve addition and subtraction problems up to 10. Kids build essential mental math problem-solving skills.
Cut & Solve – Number Bonds #5
Cut & Solve – Number Bonds #6
Kids look at six addition up to 5 problems and determine is it true or false. Kids cut and paste the equation into the correct column.
Cut & Solve – Number Bonds #6
Extending Number Bonds
Number bonds can be used to help children develop number fluency and mental math strategies. These skills are beneficial when working with numbers larger than ten. Number fluency goes beyond memorization and requires children to have an understanding of numbers. They can explain how they arrive at answers, demonstrate flexible approaches, and use efficient strategies. Mental math allows children to perform math “in their head” as opposed to working out questions on paper, using manipulatives, or using a calculator.
An example of using number bonds to find the answer to questions using larger numbers is 191+70. 70 can be broken down into 9 and 61. The 191 from the question can be added to the 9 to make 200. 200 is a relatively easy number to work with. Now you can simply add 200 to 61 to arrive at the answer of 261. Before children can do this in their heads, they may need to see it represented in number bonds.
Introducing number bonds during early grades can help children explore numbers and understand their relationships to ten. Number bonds can help teach counting, addition, and subtraction. As children progress, number bonds can be a useful strategy for working with larger numbers, including manipulating numbers using mental math strategies.
References
See the research that supports the number bonds strategy: Counting & Number Bonds: Math Games for Early Learners
LEARNING MATERIALS TO MEET EVERY CHILD’S NEEDS
Here, at PrimaryLearning.Org, we tend to deliver the best-differentiated learning materials to K-2 students. Our resources can be easily incorporated into multisensory lessons to meet every child’s needs, whether s/he is a visual, kinesthetic, or auditory learner.